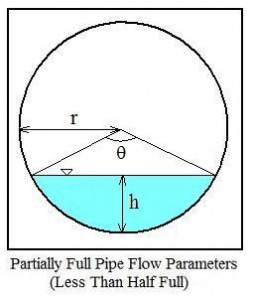
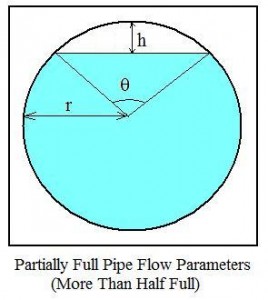
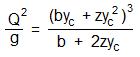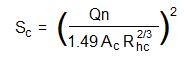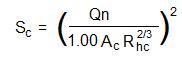Tag Archives: manning roughness coefficient

Manning Equation Open Channel Flow Calculator Excel Spreadsheets

Posted on by
Reply

Where to Find a Manning Equation Open Channel Flow Calculator Spreadsheet

To obtain a Manning equation open channel flow calculator excel spreadsheet, click here to visit our spreadsheet store.  Why use online calculators or make open channel flow/Manning Equation calculations by hand when you can buy a variety of Manning equation open channel flow calculator excel spreadsheets or spreadsheet packages for prices ranging from $6.95 to $27.95?  Read on for information about Excel spreadsheets that can be used as a Manning equation open channel flow calculator.

picture for a Manning equation open channel flow calculatorAn excel spreadsheet can conveniently be used as a Manning equation open channel flow calculator.  The Manning equation can be used for water flow rate calculations in either natural or man made open channels.  Uniform open channel flow calculations with the Manning equation use the channel slope, hydraulic radius,  flow depth, flow rate, and Manning roughness coefficient.   Image Credit: geograph.org.uk

Uniform Flow for a Manning Equation Open Channel Flow Excel Spreadsheet

Diagram for a Manning Equation Open Channel Flow Calculator Spreadsheet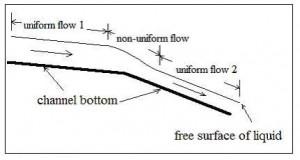Open channel flow may be either uniform flow or nonuniform flow, as illustrated in the diagram at the left.  For uniform flow in an open channel, there is always a constant volumetric flow of liquid through a reach of channel with a constant bottom slope, surface roughness, and hydraulic radius (that is constant channel size and shape).  For the constant channel conditions described, the water will flow at a constant depth (usually called the normal depth) for the  particular volumetric flow rate and channel conditions. The diagram above shows a stretch of uniform open channel flow, followed by a change in bottom slope that causes non-uniform flow, followed by another reach of uniform open channel flow.  The Manning Equation, which will be discussed in the next section, can be used only for uniform open channel flow.

Equation and Parameters for a Manning Equation Open Channel Flow Calculator Excel Spreadsheet

The Manning Equation is:

Q = (1.49/n)A(R2/3)(S1/2) for the U.S. units shown below, and it is:

Q = (1.0/n)A(R2/3)(S1/2) for the S.I. units shown below.

  • Q is the volumetric water flow rate in the reach of channel (ft3/sec for U.S.) (m3/s for S.I.)
  • A is the cross-sectional area of flow  (ft2for U.S.) (m2for S.I.)
  • P is the wetted perimeter of the flow  (ft for U.S.)  (m for S.I.)
  • R is the hydraulic radius, which equalsA/P(ft for U.S.) (m for S.I.)
  • S is the bottom slope of the channel, (dimensionless or ft/ft -U.S. & m/m – S.I.)
  • n is the empirical Manning roughness coefficient, which is dimensionless

The equation V = Q/A, a definition for average flow velocity, can be used to express the Manning Equation in terms of average flow velocity,V, instead of flow rate,Q, as follows:

V = (1.49/n)(R2/3)(S1/2) for U.S. units with V expressed in ft/sec.

Or V = (1.0/n)(R2/3)(S1/2) for S.I. units with V expressed in m/s.

It should be noted that the Manning Equation is an empirical equation.  The U.S. units must be just as shown above for use in the equation with the constant 1.49 and the S.I. units must be just as shown above for use in the equation with the constant 1.0.

The Manning Roughness Coefficient for a Manning Equation Open Channel Flow Calculator Excel Spreadsheet

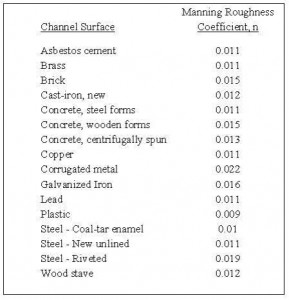Manning Equation Open Channel Flow Calculator Manning Roughness CoefficientsAll calculations with the Manning equation (except for experimental determination of n) require a value for the Manning roughness coefficient, n, for the channel surface.  This coefficient, n, is an experimentally determined constant that depends upon the nature of the channel and its surface.  Smoother surfaces have generally lower Manning roughness coefficient values and rougher surfaces have higher values. Many handbooks, textbooks and online sources have tables that give values of n for different natural and man made channel types and surfaces. The table at the right gives values of the Manning roughness coefficient for several common open channel flow surfaces for use in a Manning equation open channel flow calculator excel spreadsheet.

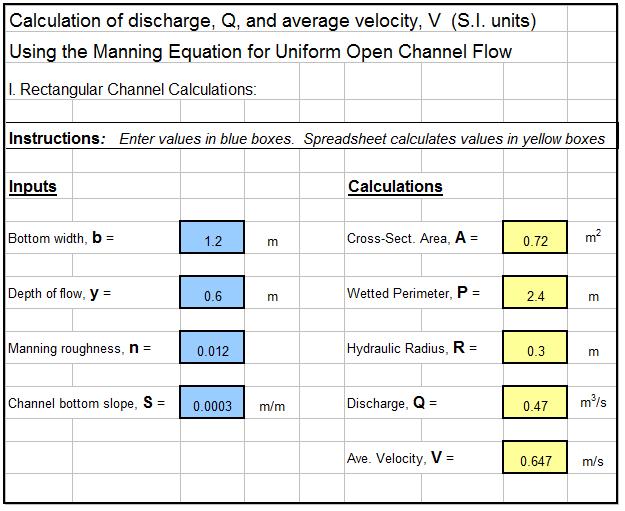
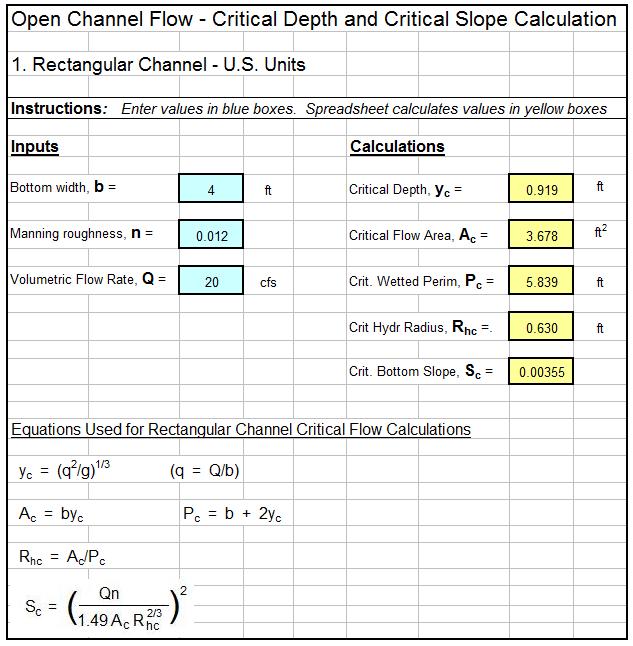
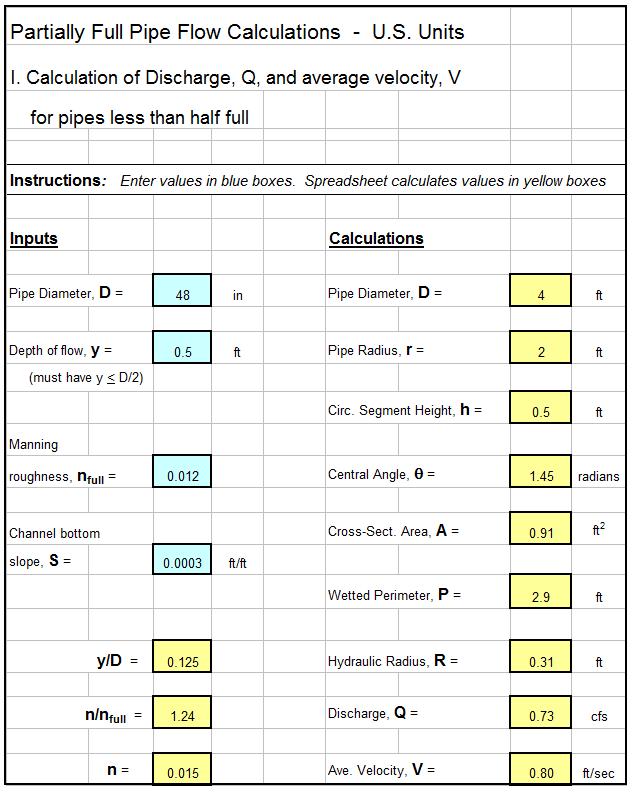
Example Manning Equation Open Channel Flow Excel Spreadsheet

The Manning equation open channel flow calculator excel spreadsheet shown in the image below can be used to calculate flow rate and average velocity in a rectangular open channel with specified channel width, bottom slope, & Manning roughness, along with the flow rate through the channel.  This Excel spreadsheet and others for Manning equation open channel flow calculations for rectangular, trapezoidal or triangular channels, in either U.S. or S.I. units are available for very reasonable prices in our spreadsheet store.

Manning Equation Open Channel Flow Calculator Excel Spreadsheet

References

1. Bengtson, Harlan H., Open Channel Flow I – The Manning Equation and Uniform Flow, an online, continuing education course for PDH credit.

2. U.S. Dept. of the Interior, Bureau of Reclamation, 2001 revised, 1997 third edition, Water Measurement Manual.

3. Chow, V. T., Open Channel Hydraulics, New York: McGraw-Hill, 1959.

4.  Bengtson, Harlan H., “Manning Equation Open Channel Flow Excel Spreadsheets,”  an online blog article, 2012.

5. Bengtson, Harlan H., “The Manning Equation for Open Channel Flow Calculations“, available as an Amazon Kindle e-book and as a paperback.

Flow Through Annulus Calculator Excel Spreadsheet

Posted on by
Reply

Where to Find an Excel Spreadsheet Flow Through Annulus Calculator

For an Excel spreadsheet liquid flow through annulus calculator, click here to visit our spreadsheet store.  Look in the “Non-Circular Duct flow Calculations” category.  Obtain a convenient, easy to use spreadsheet liquid flow through annulus calculator at a reasonable price. Read on for information about the use of Excel spreadsheets to calculate pressure drop or liquid flow rate for annulus flow.

Friction Factor-Pipe Flow Background for a Liquid Flow Through Annulus Calculator

A liquid flow through annulus calculator spreadsheet uses calculations that are very similar to those for flow through a pipe.  The main difference is use of the hydraulic diameter for flow through an annulus in place of the pipe diameter as used for pipe flow.  For details of pipe flow calculations, see the article, “Friction Factor/Pipe Flow Calculations with Excel Spreadsheets.”

Calculation of the Hydraulic Diameter for a Liquid Flow Through Annulus Calculator

The general definition of hydraulic diameter for flow through a non-circular cross-section is:                               DH = 4(A/P),    where:

  • DH is the hydraulic diameter in ft (m for S.I. units)
  • A is the cross-sectional area of flow in sq ft (sq m for S.I. units)
  • P is the wetted perimeter in ft (m for S.I. units)

For a flow through annulus calculator:

  • A = (π/4)(Do2 –  Di2)
  • P  =  π(Do + Di)

Where Do is the inside diameter of the outer pipe and Di is the outside diameter of the inner pipe.  Substituting for A and P in the definition of  DH and simplifying gives:

DH =  Do – Di

Equations for the Liquid Flow Through Annulus Calculator

The Darcy Weisbach equation for flow in an annulus is:  hL = f(L/DH)(V2/2g), with the parameters in the equation as follows: hL is the frictional head loss for flow of a liquid at average velocity, V, through an annulus of length, L, and hydraulic diameter, DH .  The Reynolds number for the flow (Re) and the relative roughness of the pipe (Manning roughness coefficient /pipe diameter, ε/D) are needed to get a value for the friction factor, f.  The Moody friction factor diagram and equations for calculating the friction factor, f, are presented and discussed in the article, “Friction Factor/Pipe Flow Calculations with Excel Spreadsheets.”

Spreadsheets for the Liquid Flow Through Annulus Calculator

The Excel spreadsheet screenshot below shows a liquid flow through annulus calculator spreadsheet for calculation of the head loss and frictional pressure drop for flow of a liquid through an annulus.  Based on the input values for the annulus diameters and length as well as liquid flow rate and properties, the spreadsheet will calculate the head loss and frictional pressure drop.

For low cost, easy to use spreadsheets to make these calculations as well as similar calculations for liquid flow in an annulus or for pipe flow calculations, in S.I. or U.S. units, click here to visit our spreadsheet store.

liquid flow through annulus calculator spreadsheetReferences

1.  Munson, B. R., Young, D. F., & Okiishi, T. H., Fundamentals of Fluid Mechanics, 4th Ed., New York: John Wiley and Sons, Inc, 2002.

2. Bengtson, H.H., Pipe Flow/Friction Factor Calculations with Excel, an online continuing education course for Professional Engineers.

3.  Bengtson, Harlan H.,  Advantages of Spreadsheets for Pipe Flow/Friction Factor Calculations,  an e-book available through Amazon.com.

Critical Depth Open Channel Flow Spreadsheet

Posted on by
2

Where to Find a Critical Depth Open Channel Flow Spreadsheet

To obtain a critical depth open channel flow spreadsheet for calculating critical depth and/or critical slope for open channel flow, click here to visit our spreadsheet store.  Read on for information about the use of a critical depth open channel flow spreadsheet for critical depth and critical slope calculations.

The Froude Number and Critical, Subcritical and Supercritical Flow

Any particular example of open channel flow will be critical, subcritical, or supercritical flow.  In general, supercritical flow is characterized by high liquid velocity and shallow flow, while subcritical flow is characterized by low liquid velocity and relatively deep flow.  Critical flow is the dividing line flow condition between subcritical and supercritical flow.

The Froude number is a dimensionless number for open channel flow that provides information on whether a given flow is subcritical, supercritical or critical flow.  The Froude number is defined to be:  Fr = V/(gL)1/2 , where V is the average velocity, g is the acceleration due to gravity, and L is a characteristic length for the particular type of open channel flow.  For flow in a rectangular channel:  Fr = V/(gy)1/2 ,   where y is the depth of flow.  For flow in an open channel with a shape other than rectangular:  Fr = V/[g(A/B)]1/2 , where A is the cross-sectional area of flow, and B is the surface width.

The value of the Froude number for a particular open channel flow situation gives the following information:

  • For Fr < 1, the flow is subcritical
  • For Fr = 1, the flow is critical
  • For Fr > 1, the flow is supercritical

Calculation of Critical Depth

It is sometimes necessary to know the critical depth for a particular open channel flow situation.  This type of calculation can be done using the fact that Fr = 1 for critical flow.  It is quite straightforward for flow in a rectangular channel and a bit more difficult, but still manageable for flow in a non-rectangular channel.

For flow in a rectangular channel (using subscript c for critical flow conditions), Fr = 1 becomes:   Vc/(gyc)1/2 = 1.  Substituting Vc =  Q/Ac =  Q/byc and  q = Q/b  (where b = the width of the rectangular channel), and solving for yc gives the following equation for critical depth: yc =  (q2/g)1/3.   Thus, the critical depth can be calculated for a specified flow rate and rectangular channel width.

For flow in a trapezoidal channel, Fr = 1 becomes:  Vc/[g(A/B)c]1/2 = 1.  Substituting the equation above for Vc together with Ac =  yc(b + zyc)    and   Bc =  b  +  zyc2 leads to the following equation, which can be solved by an iterative process to find the critical depth:

Critical Depth Open Channel Flow Spreadsheet Formula1

Calculation of Critical Slope

After the critical depth, yc ,  has been determined, the critical slope, Sc , can be calculate using the Manning equation if the Manning roughness coefficient, n, is known.  The Manning equation can be rearranged as follows for this calculation:

Critical Depth Open Channel Flow Spreadsheet Formula2Note that Rhc , the critical hydraulic radius, is given by:

Rhc =  Ac/Pc,  where Pc =  b  +  2yc(1 + z2)1/2

Note that calculation of the critical slope is the same for a rectangular channel or a trapezoidal channel, after the critical depth has been determined.  The Manning equation is a dimensional equation, in which the following units must be used:  Q is in cfs, Ac is in ft2, Rhc is in ft, and Sc and n are dimensionless.

Calculations in S.I. Units

The equations for calculation of critical depth are the same for either U.S. or S.I. units.  All of the equations are dimensionally consistent, so it is just necessary to be sure that an internally consistent set of units is used.  For calculation of the critical slope, the S.I. version of the Manning equation must be used, giving:

Critical Depth Open Channel Flow Spreadsheet Formula4In this equation, the following units must be used:  Q is in m3/s, Ac is in m2, Rhc is in m, and Sc and n are dimensionless.

A Critical Depth Open Channel Flow Spreadsheet Screenshot

The critical depth open channel flow spreadsheet template shown below can be used to calculate the critical depth and critical slope for a rectangular channel with specified flow rate, bottom width, and Manning roughness coefficient.  Why bother to make these calculations by hand?  This Excel spreadsheet and others with similar calculations for a trapezoidal channel are available in either U.S. or S.I. units at a very low cost in our spreadsheet store.

Critical Depth Open Channel Flow Spreadsheet Screenshot

References

1. Munson, B. R., Young, D. F., & Okiishi, T. H., Fundamentals of Fluid Mechanics, 4th Ed., New York: John Wiley and Sons, Inc, 2002.

2. Chow, V. T., Open Channel Hydraulics, New York: McGraw-Hill, 1959.

3. Bengtson, Harlan H. Open Channel Flow II – Hydraulic Jumps and Supercritical and Nonuniform Flow – An online, continuing education course for PDH credit.

 

 

 

Partially Full Pipe Flow Calculator with Excel Spreadsheets

Posted on by

Where to Find Partially Full Pipe Flow Calculator Spreadsheets

To obtain Excel spreadsheets for partially full pipe flow calculations, click here to visit our spreadsheet store  for partially full pipe flow calculator spreadsheets. Read on for information about Excel spreadsheets that can be used as a partially full pipe flow calculator.

The Manning equation can be used for flow in a pipe that is partially full, because the flow will be due to gravity rather than pressure.  the Manning equation [Q = (1.49/n)A(R2/3)(S1/2) for (U.S. units) or Q = (1.0/n)A(R2/3)(S1/2) for (S.I. units)] applies if the flow is uniform flow  For background on the Manning equation and open channel flow and the conditions for uniform flow, see the article, “Manning Equation/Open Channel Flow Calculations with Excel Spreadsheets.”

Graph for use with a partially full pipe flow calculatorDirect use of the Manning equation as a partially full pipe flow calculator, isn’t easy, however, because of the rather complicated set of equations for the area of flow and wetted perimeter for partially full pipe flow.  There is no simple equation for hydraulic radius as a function of flow depth and pipe diameter.  As a result graphs of Q/Qfull and V/Vfull vs y/D, like the one shown at the left are commonly used for partially full pipe flow calculations.  The parameters, Q and V in this graph are flow rate an velocity at a flow depth of y in a pipe of diameter D.  Qfull and Vfull can be conveniently calculated using the Manning equation, because the hydraulic radius for a circular pipe flowing full is simply D/4.

With the use of Excel formulas in an Excel spreadsheet, however, the rather inconvenient equations for area and wetted perimeter in partially full pipe flow become much easier to work with.  The calculations are complicated a bit by the need to consider the Manning roughness coefficient to be variable with depth of flow as discussed in the next section.

Is the Manning Roughness Coefficient Variable for Partially Full Pipe Flow Calculations?

Using the geometric/trigonometric equations discussed in the next couple of sections, it is relatively easy to calculate the cross-sectional area, wetted perimeter, and hydraulic radius for partially full pipe flow  with any specified pipe diameter and depth of flow.  If the pipe slope and Manning roughness coefficient are known, then it should be easy to calculate flow rate and velocity for the given depth of flow using the Manning Equation                             [Q = (1.49/n)A(R2/3)(S1/2)], right?   No, wrong!  As long ago as the middle of the twentieth century, it had been observed that measured flow rates in partially full pipe flow aren’t the same as those calculated as just described.  In a 1946 journal article (ref #1 below), T. R. Camp presented a method for improving the agreement between measured and calculated values for partially full pipe flow.  The method developed by Camp consisted of using a variation in Manning roughness coefficient with depth of flow as shown in the graph above.

Although this variation in Manning roughness due to depth of flow doesn’t make sense intuitively, it does work.  It is well to keep in mind that the Manning equation is an empirical equation, derived by correlating experimental results, rather than being theoretically derived.  The Manning equation was developed for flow in open channels with rectangular, trapezoidal, and similar cross-sections.  It works very well for those applications using a constant value for the Manning roughness coefficient, n.  Better agreement with experimental measurements is obtained for partially full pipe flow, however, by using the variation in Manning roughness coefficient developed by Camp and shown in the diagram above.

The graph developed by Camp and shown above appears in several publications of the American Society of Civil Engineers, the Water Pollution Control Federation, and the Water Environment Federation from 1969 through 1992, as well as in many environmental engineering textbooks (see reference list at the end of this article).  You should beware, however that there are several online calculators and websites with equations for making partially full pipe flow calculations using the Manning equation with constant Manning roughness coefficient, n.  The equations and Excel spreadsheets presented and discussed in this article use the variation in n that was developed by T.R. Camp.

Excel Spreadsheet/Partially Full Pipe Flow Calculator for Pipe Less than Half Full

Diagram to for Partially Full Pipe Flow CalculatorThe parameters used in partially full pipe flow calculations with the pipe less than half full are shown in the diagram at the right.  K is the circular segment area; S is the circular segment arc length; h is the circular segment height; r is the radius of the pipe; and θ is the central angle.

The equations below are those used, together with the Manning equation and Q = VA, in the partially full pipe flow calculator (Excel spreadsheet) for flow depth less than pipe radius, as shown below.

  • h = y
  • θ = 2 arccos[ (r – h)/r ]
  • A = K = r2(θ – sinθ)/2
  • P = S = rθ

The equations to calculate n/nfull, in terms of y/D for y < D/2 are as follows

  • n/nfull = 1 + (y/D)(1/3) for 0 < y/D < 0.03
  • n/nfull = 1.1 + (y/D – 0.03)(12/7) for 0.03 < y/D < 0.1
  • n/nfull = 1.22 + (y/D – 0.1)(0.6) for 0.1 < y/D < 0.2
  • n/nfull = 1.29 for 0.2 < y/D < 0.3
  • n/nfull = 1.29 – (y/D – 0.3)(0.2) for 0.3 < y/D < 0.5

The Excel template shown below can be used as a partially full pipe flow calculator to calculate the pipe flow rate, Q, and velocity, V, for specified values of pipe diameter, D, flow depth, y, Manning roughness for full pipe flow, nfull; and bottom slope, S, for cases where the depth of flow is less than the pipe radius.  This Excel spreadsheet and others for partially full pipe flow calculations are available in either U.S. or S.I. units at a very low cost in our spreadsheet store.

screenshot of partially full pipe flow calculator spreadsheet

Excel Spreadsheet/Partially Full Pipe Flow Calculator for Pipe More than Half Full

The parameters used in partially full pipe flow calculations with the pipe more than half full are shown in the diagram at the right.  K is the circular segment area; S is the circular segment arc length; h is the circular segment height; r is the radius of the pipe; and θ is the central angle.

The equations below are those used, together with the Manning equation and Q = VA, in the partially full pipe flow calculator (Excel spreadsheet) for flow depth more than pipe radius, as shown below.

  • h = 2r – y
  • θ = 2 arccos[ (r – h)/r ]
  • A = πr2 – K = πr2 – r2(θ – sinθ)/2
  • P = 2πr – S = 2πr – rθ

The equation used for n/nfull for 0.5 < y//D < 1 is: n/nfull = 1.25 – [(y/D – 0.5)/2]

An Excel spreadsheet like the one shown above for less than half full flow, and others for partially full pipe flow calculations, are available in either U.S. or S.I. units at a very low cost at www.engineeringexceltemplates.com.

References

1. Bengtson, Harlan H.,  Uniform Open Channel Flow and The Manning Equation, an online, continuing education course for PDH credit.

2. Camp, T.R., “Design of Sewers to Facilitate Flow,” Sewage Works Journal, 18 (3), 1946

3. Chow, V. T., Open Channel Hydraulics, New York: McGraw-Hill, 1959.

4. Steel, E.W. & McGhee, T.J., Water Supply and Sewerage, 5th Ed., New York, McGraw-Hill Book Company, 1979

5.  ASCE, 1969. Design and Construction of Sanitary and Storm Sewers, NY

6. Bengtson, H.H., “Manning Equation Partially Filled Circular Pipes,”  An online blog article

7. Bengtson, H.H., “Partially Full Pipe Flow Calculations with Spreadsheets“, available as an Amazon Kindle e-book and as a paperback.